I was thinking about how you calculate percent solutions the other day when discussing Glacial Acetic Acid mixtures to make stop bath. I knew I had seen an easy method, but I could not remember how it worked. Certainly it is fine to just follow directions, but what if you want to know how the process works, or you need to calculate some dilution other than the norm? I thought the equation was called the ‘X’ System or something similar. A search on the Internet did not turn up what I was looking for though. Seems things always come to me when I take a nap. . . this time I had to sleep on this one for several days before it came to me.
The simple procedure for calculating percent dilutions is called the Criss-Cross method and is really easy, if you can remember how it works. Once I had the right description, it was easy to find more information, and it is really quite simple.
Here is the Criss-Cross formula;
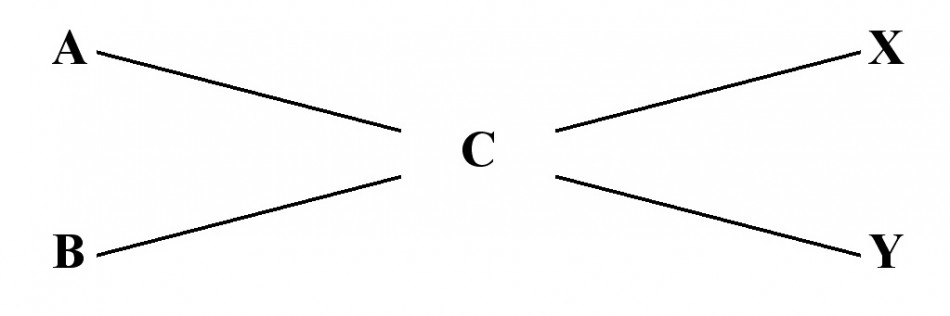
To work the Criss-Cross formula do the following:
A = the % dilution of the solution to be diluted
B = the % dilution of the diluting solution (for Water this value is Zero)
C = the % dilution desired
X = C – B
Y = A – C
Diluting X parts of A with Y parts of B will yield a % solution equal to C.
I know it all sounds complicated and it really is much more difficult to explain than it is to actually work the problem. So, lets go through an example that hopefully will make it more understandable. Most all agree that an acid stop bath should be somewhere between a 1-2% dilution of Acetic Acid in water. I have always used a 1% solution and that is what we still use today. For example, let’s dilute 28% Acetic Acid stock to a 1% mixture for stop bath. Plug in the correct values, then perform the calculation. Enter 28% for term A, 0 for term B, 1% for term C, then perform the calculation.

You will find that if you mix 1 unit of 28% Acid with 27 units of Water you will get a 1% solution for your stop bath. Remember, we are working with ratios and the Units can be anything desired, as long as they are the same Units. It could be a mixture of 1:27 ounces, gallons, milliliters, liters, whatever units you desire. You can change the values of X and Y if you want. Just keep in mind that you have to change both Units by the same amount. If you multiply the ratio of 1:27 by 2, you would have a ratio of 2:54 Units. You could also divide the Units for smaller volumes.
For the sake of a working example, if you multiply the ratio of 1:27 in the example above by 40, you get a ratio of 40:1,080. This is how I dilute 28% Acetic Acid to a 1% solution for paper stop bath. I use even numbers of 40:1,000 milliliters. Plenty close enough for photography.
Hope this helps. . . it is not difficult if you can remember the formula.
JB

